

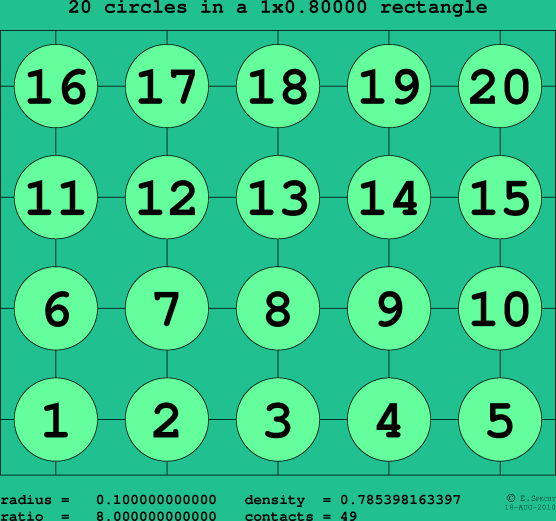
Apart from physical experiment, it is prima facie a more economical use of space-the arrangement is more compact-with three circles crowded into a corner (second position) than with only two along one side and the corners unoccupied (first position). Input the rectangle inside dimensions - height and width and the circles outside diameters. If the rectangle were changed to a square with side $13.96$, the quarters would fit symmetrically about the diagonal and be more compact than in the original arrangement, since $$13.96^2<14.33\times13.90$$īut even without altering the rectangle, the circles have room to move into the second position. quarters, and was easily able to rotate them into the position shown in the next figure.
I made a wooden frame of this shape, sized just to hold a regular pentagon array of five U.S. Hence the rectangle that encompasses the two circles is of. Having a good gist of the properties of rectangle and square helps in getting the answer.For unit circle, the length of the rectangle is the pentagon diagonal + $2$, that is $$2\cdot \frac=1.031$$ The two equal circles are of 12 cm radius each, because their center to center distance is 12 cm. Videos on YouTube, problem from the 2017 GCSE exam. Thus the area of the rectangle is: (4 r) r (2 + 3) 4 r2 (2 + 3) Sources. We then add 2 radius lengths to get the entire side length, which is then r (2 + 3). The square BLMN inscribes two circles and rectangle as shown in the figure, if the radius of the smaller circle is 7 cm, and the radius of the larger. These probelems can easily by solved using the pythagorean theorem, see pictures below. If we connect the centers of the 3 circles, we get an equilateral triangle with a side length of 2 r. Note: Whenever we face such types of problems the key concept is simply to have a diagrammatic representation of the information provided in the question as it helps to understand the basic geometry of the figure. Distance between center of two circles connected by one tangent. You may use computer integration where the area is divided into many small rectangle and calculating the sum of them, or just use closed form here. So the rectangle of maximum area inscribed in a circle is a square. Two circles are inscribed in a rectangle as shown above. The area can be calculate by integrating the circle equation y sqrt a2 - (x-h)2 + k where a is radius, (h,k) is circle center, to find the area under curve. Hence x = y $ = r\sqrt 2 $ thus it forms a square with maximum area. Now in triangle BCD apply Pythagoras theorem we have, Hence let the sides of the rectangle be x and y respectively as shown in figure. Since the rectangle has all the four coordinates inscribed on the circumference of the circle. The diagonal of the rectangle will be the diameter of the circle.Īs we know diameter (d) is twice the radius. Divide just one of the sectors into two equal parts. Then, find the area of one half the circle, a semi-circle, by dividing your final answer by 2.
#Two circles in rectangle full#
Let ABCD be the rectangle inscribed in the circle with center O and radius (r). The area of a full circle is 50.24 square inches.


 0 kommentar(er)
0 kommentar(er)
